Published in: K. Martinas, L. Ropolyi, P. Szegedi (Eds.)
THERMODYNAMICS: HISTORY AND PHILOSOPHY. FACTS, TRENDS, DEBATES. World Scientific Publishing Co., 1991 (ISBN 981-02-0464-7), p. 492-507.
The paper deals mainly with relations Gibbs' work to the problems which chemical thermo¬dynamics met in course of its development. In particular, 'pre-thermodynamic’ and 'post-thermodynamic’ models are considered.
We begin with the well-known assertion that Gibbs was a founder of chemical thermodynamics. One might be astonished looking at the scheme representing the origin of the chemical thermodynamics. Gibbs' works are not presented there, and this is not an occasion, since chemical thermodynamics, just as a chemical discipline, arose really beyond his works - as far as it concerns the arising, not the further development.
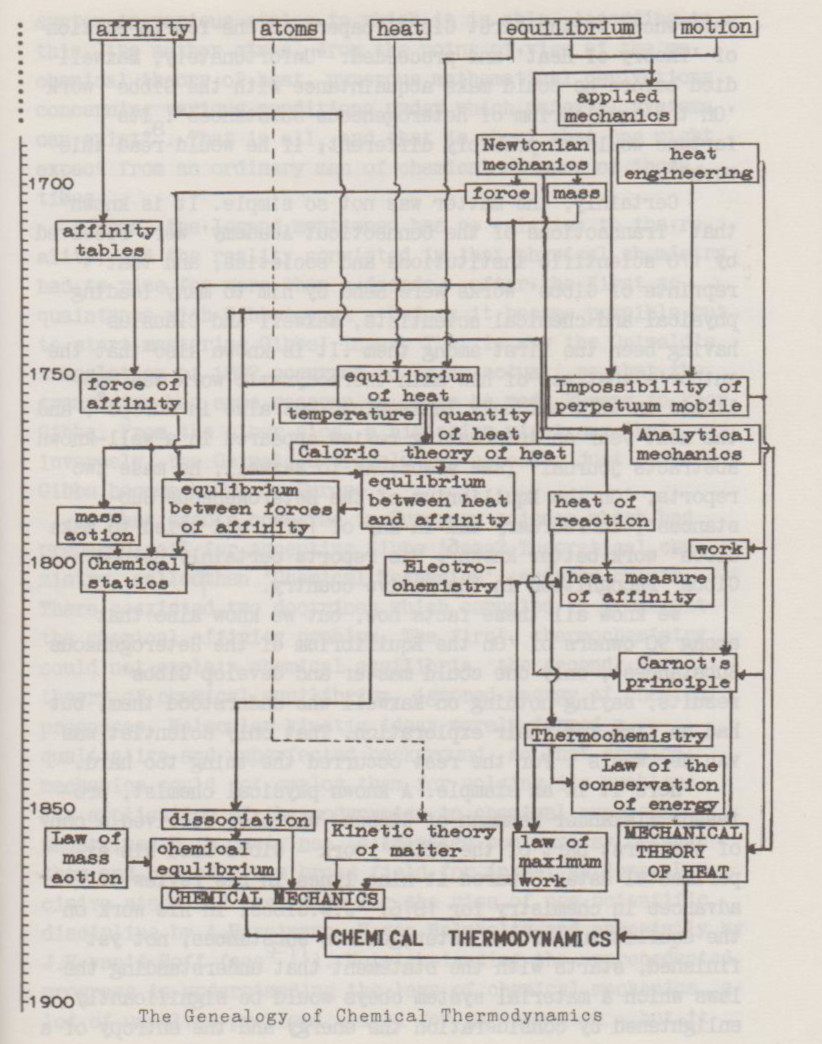
There existed, almost until now, a stable legend that it occurred simply because Gibbs' works stayed unknown before their German translation was published by Wilh. Ostwald in 1892. E.g. V.Semenchenko, the editor of Russian translation of Gibbs thermodynamic works, marked Maxwell's references to the first Glbbs' papers in the fourth edition of 'Theory of Heat' and proceeded: "Unfortunately, Maxwell died before he could make acquaintance with the Glbbs’ 'On the equilibrium of heterogeneous substances'. Its fortune would be probably different, if he would read this work"[1].
Certainly, the matter was not so simple. It is known [2]
that 'Transactions of the Connecticut Academy' were received by 170 scientific institutions and societies, and that reprints of Glbbs' works were send by him to many leading physical and chemical scientists, Maxwell and Clausius having been the first among them. It is known also that the author's abstract of his main thermodynamic work was published in 1879 not only In America, but also in Europe [3], and the next year an interesting review appeared in a well-known abstracts journal [4] (see also [5]).As to Maxwell, he made two reports, 'On the Equilibrium of the Heterogeneous Substances', in February and In May of 1876, and tried to make Gibbs' work better known [6]. His reports certainly helped Gibbs' recognition in the native country.
We know all these facts now, but we know also that among 90 owners of 'On the Equilibrium of the Heterogeneous Substances', only one could master and develop Gibbs' results, saying nothing on Maxwell who understood them, but had no time for their exploration. That only scientist was van der Waals [7]. For the rest occurred the thing too hard.
Here it is an example. A known physical chemist, professor Alexander Naumann of Gissen, who also received a copy of the first part of the Gibbs' work - Gibbs used his experimental data - spared it nine lines in his review of advances in chemistry for 1876: "J. W. Gibbs, in his work on the equilibrium of the heterogeneous substances, not yet finished, starts with the statement that understanding the laws which a material system obeys would be significantly enlightened by consideration the energy and the entropy of a system in various states to which it is able. According to this, the author gives, from the point of view of the mechanical theory of heat, numerous mathematical derivations concerning various conditions under which material systems can exist" [8]. That is all, and that is about what one might expect from an ordinary man of chemical science of those times.
Thus, the legend mentioned has no relation to the reality, and the reality consisted in that physical chemistry had to rise for more than a decade - after the first acquaintance with Gibbs’ work - before it became possible but to start mastering Gibbs' ideas. That is why the Ostwald's translation of 1892 occurred to be so actual, so that the translator had some reasons to claim he made Europe to know Gibbs. From the other side, a historian might say that, inversely, the Ostwald's translation appeared just because Gibbs became known in Europe.
What events occurred in European science which had prepared soil for accepting Gibbs ideas? Theoretical chemistry, called then 'Chemical Mechanics', endured crisis. There coexisted two doctrines which competed in treatment the chemical affinity problem. The first, thermochemistry, could not explain chemical equilibria, the second, the theory of chemical equilibrium, ignored energy of chemical processes. Molecular kinetic ideas merely formed a qualitative and unperfected background, so that chemical mechanics could not employ them for solving its problems. The application of thermodynamics to chemical problems underpined both doctrines, eliminated controversy between them and opened a new broad field for investigations. Decisive steps had been made for the rise of new scientific discipline by A. Horstmann, H. von Helmholtz.and especially by J. H. van't Hoff (see [9-11]). Notwithstanding the unprecedented progress in understanding the laws of chemical mechanics, a lot of unsolved problems stayed for further work - but it was discovered later, as we clearly realize at present, that most of these problems had been solved, or formulated, by Gibbs.
Let us shortly recollect, by mere enumeration, what Gibbs had done.
First, he created the general method consisting in use of thermodynamic potentials simultaneously both as characteristic functions and as functions immediately giving conditions of the equilibrium.
Second, he expanded the method into chemistry by introducing notions of phases and of components, and by introducing chemical potentials to take into account the role of individual substances in the equilibrium.
At last, he gave, on the said foundation, the theory for main cases of equilibria in chemistry, that is, of chemical and phase equilibria, of stable and unstable states, of equilibria including capillary and electric forces.
The first of above points deserves some short comments. The most important of them is, as follows. It was beginning from Gibbs, that the two propositions, on the existence of entropy and of its increase in irreversible processes,- traditionally combined in what is called the second principle of thermodynamics - could be discerned. Gibbs was the first to employ explicitly the both propositions. It is this feature, that makes the Gibbs’ method the most powerful in thermodynamics in general, and in chemical thermodynamics in particular, since other respects of the Gibbs’ analytic method essentially coincide with what had been already done by Clausius and Massieu [12].
Another short remark concerns the principle of equilibrium: its genetic relation to analytic mechanics was re¬peatedly pointed out by those, who investigated Gibbs’ work, but the statement had been claimed by Gibbs himself when he underlined that his criteria of equilibrium, expressed via either energy or free energy, may "both be regarded as extensions of the criterion employed in ordinary statics to the more general case of a thermodynamic system" [13]. (It is to the point, to note here that mechanics had a very important part not only in the Glbbs' system, but also in the formation of chemical thermodynamics at all; it could be even seen from the scientific language of those times: its ‘parents' were the mechanical theory of heat and chemical mechanics).
The last what will be recalled about the methods is his extraordinary contribution to geometric methods in thermodynamics consisting in using thermodynamic functions as coordinates in state diagrams. (It is to note that the diagram entropy-temperature had been at first plotted by Belgian engineer Th. Belpair in 1872 [14]).
Gibbs’ second great contribution was his way of expanding thermodynamics on chemistry.
Thermodynamics was at first exactly what its name expressed, that is, the theory of the motive power of heat. As Maxwell wrote, "we define thermodynamics ... as the investigation of the dynamical and thermal properties of bodies, deduced entirely from what are called the First and Second Laws of Thermodynamics without any hypotheses as to molecular constitution of bodies" [15].
The broadest generalizations underlying this 'primary' thermodynamics made, however, possible, moreover, made logically inevitable the expansion of deductions on other fields of science. Next of these was chemistry.
To make the expansion possible, some additional information was needed about new objects destined henceforth to thermodynamic investigation. This information may be called a 'pre-thermodynamic model' of the object. For chemistry were such models, first of all, phases and components, and further, critical phases, interfaces, semipermeable membranes, charged components. All these are very general concepts on the structure of chemical systems. They are taken from the experience and form the material for thermodynamics proper.
An apt remark might be cited as an example; it belongs to G. Tamman: "One cannot expect the pure thermodynamics would be able to answer, if both kinds of diagrams, with end points and without them, do exist indeed. The pure thermodynamics is so far from reality that it does not even know if state diagrams do exist at all" [16].
It is combining with the pre-thermodynamic informatics, that transformed new-born thermodynamics into a broad experimental science, and one of its chapters was just chemical thermodynamics.
This important fact becomes clear just when one considers Gibbs' work, since it is here, that we can see the logic of development and problems of axiomatlcs in thermodynamics.
As to other branch of chemical thermodynamics that had originated from chemical facts and difficulties, it did not know this problem at all, the logical structure of thermodynamics was quite of no Importance here. Van't Hoff took from thermodynamics just what he needed for consideration chemical facts. 'Pre-thermodynamics' was for him the real experience, the matter he immediately worked at, because his object was chemistry, not thermodynamics. This displayed in particular in van't Hoff's way of taking into account roles of individual substances: his foundation was the chemical fact that semipermeable membranes existed; he was alien to abstract chemical potentials.
It is worthy of saying a few words about these functions. 'Potentials' are the central notion in the Gibbs' system of chemical thermodynamics, and one might guess they were his beloved creation. A good half of the well-known letter to The American Academy of Arts and Sciences where Gibbs had characterized his work is devoted to potentials. He wrote in particular: "...it is the office of theoretical investigation to give the form in which the results of experiments may be expressed. In the present case we are led to certain functions which play the principal part in determining the behavior of matter in respect to chemical equilibrium. The forms of these functions, however, remain to be determined by experiment, and here we meet the greatest difficulties, and find an inexhaustible field of labor" [17].
It was extremely important for him, to show potentials made possible to get the same results that had been already obtained by van't Hoff, and Raoult, and Helmholtz. His striving for demonstration the effectiveness of these functions clearly manifested in his letters to O. Lodge [18] and to W. D. Bancroft [19]. (It would be remembered that it was Bancroft, who had introduced the term 'chemical potential' as well as the name 'phase rule'). Let us quote two his phrases on potentials: "I cannot say that the term has been adopted by physicists. It has, however, received the un¬qualified commandation of Professor Maxwell (...see his lecture ... in the science conferences at South Kensington, 1876); and I do not see how we can do very well without the idea in certain kinds of investigation" [18]. These words seem to show an uneasy feeling arising from the lack of understanding his ideas by contemporaries.
The opposition: 'potentials against semipermeable membranes' reflected the dualism of the chemical thermodynamics origin that has already been seen from its genealogy: from the one side, in chemistry, attempts were made to draw thermodynamics for solving chemical problems, from the other side, in physics, attempts were made to expand the field of deductions especially on chemistry. Such birth-marks were always stamped on physical chemistry in general. The case of chemical thermodynamics is the most expressive, however: it was due to appearance of highly perfect and completed system created by Glbbs, that chemical thermodynamics had been really divided into two different branches which were conventionally designated as 'pre-glbbsian' and 'gibbsian' chemical thermodynamics [5]. Their amalgamation occurred continuously during 1920s-1940s. This rather late merging had its reasons; in short, the matter was that the both branches were viable enough and had their own objects, own people, and partly even own geographic boundaries. For some details we might refer to previous publications [5, 9, 12] and pass to another aspect of the legacy of Gibbs.
The condition for thermodynamics expansion to chemistry was the adoption some generalized pre-thermodynamic models, moreover, their incorporation into thermodynamics. The last gives equations, however, which connect unknown quantities, even after the said incorporation, so that neither side of the equation could be found without some additional in¬formation, that is without what might be called 'post-thermodynamic models'. Thus, there is here the problem called 'the fecondation of thermodynamics', in the apt expression of a Russian physical chemist A. Rakovsky.
The problem had not been evident at that time. Probably, three men only – Gibbs, Guldberg and van der Waals – realized then clearly that all branches of thermodynamics - general, technical, and chemical - were at first essentially the thermodynamics of ideal systems. All the advances in equilibria calculation based on the equation PV/T = const, at first for gases and then, beginning from Horstmann and van't Hoff, for solutions. Gibbs saw this clearly and commented his own calculations, as follows: "Only in the simplest case, that of gases, have I been able to write the equation expressing such a function for a body of variable composition, and here the equation only holds with a degree of approximation corresponding to the approach of the gas to the state which we call perfect" [17].
The equation of state for ideal gases was just the first of 'post-thermodynamic models'. The second one appeared one hundred years ago: that was the van der Waals equation of state for mixtures. It is from that time, that the history of thermodynamics, both of general and of chemical, ceases to be the history of thermodynamics of ideal systems and becomes the history of overcoming the 'ideal approach', that is, becomes the history of changing the single model for a multitude of models destined to represent a multitude of real systems.
Some comments are wishful concerning the term 'model' used here. The information employed to make thermodynamic equations 'telling' can be embodied either in concrete experimental data for the system which is studied or in certain 'model' of the system, that is, in an object which replaces the system in the aspect considered. It seems reasonable to distinguish the three broad classes of models in thermodynamics which may be designated as 'physical', ‘mathematical' and 'structural' models. Physical models mean known systems that are thermodynamically similar to the system under consideration; mathematical models - they may be, in turn, divided into 'analytic’ and 'geometric' - are formulae to be included into bar thermodynamic equations, as e.g. an equation of state, and/or certain geometric figures representing the system; at last, structural models consist in some propositions as to how the system to be studied is composed. All the classes of models are interconnected, but it is obvious that we have to consider structural models as primary ones: they are necessary both to select adequate physical models, that is, systems with the same values of thermodynamic similarity criteria, and to design mathematical models.
Let us consider Gibbs’ contributions to chemical thermodynamics from this point of view.
Mathematical models would be but mentioned. It is the result of absorption the Gibbs' legacy, that the necessity of obtaining the explicit analytic expressions for the chemical potentials of components, or for the free energy of a system as a whole, had been clearly understood in chemical thermodynamics. Almost the same could be said on geometric models: Glbbs' 'geometric illustrations' in his main thermodynamic work had become a real mealstone for so called 'Geometric Thermodynamics', as the domain was coined by a Russian thermodynamist A. Mlodzeevskij. (According his de¬finition, geometric thermodynamics creates "so to say, 'a geometric story' on transformations in nature; from the other side, it establishes particular physical laws, basing on the general laws of thermodynamics, by application geometric methods" [20]). Gibbs was the first to demonstrate here the synthesis the thermodynamics with the model: he designed thermodynamic surfaces, their peculiarities having been imposed by the model. The van der Waals’ famous Ψ-surface representing behavior of binary mixtures received its name in honor of Gibbs [7].
Gibbs' interest to physical models in thermodynamics is less known. He was obviously impressed by the principle of corresponding states and intended to study 'similarity in thermodynamics' [21] - that can be seen from his posthumous notes - but had no time to realize the project. Nevertheless his principal ideas survived in the letter to C. Barus [22]. Since it had been included neither to 'The Collected Works' nor to 'A Commentary' on them, some passages are worthy of quotation.
" A good deal of work has been spent in the endeavor to find equations which shall represent the relations p,t,v for various substances. It would be very pleasant to have such an equation, but it is doubtful whether the relation can be represented by any simple formula...But it is worthy, I think, of much effort to find out whether, or with what degree of approximation, and within what limits, and with what exceptions, the actual laws for different substances are similar...And if not similar, to find how many degrees of variation there are, in addition to the three connected with the measure of temperature, pressure and volume. In other words, to find how many independent constants there are in the general formula, i.e., in a formula sufficiently general to be so called". Glbbs proposed, as the first step, to compare valuesdlogp/dlogt at critical point for various substances "We may then ask whether, or how far, these substances, which have the same limiting values dlogp/dlogt, have similar relations between p, t, and v... In this way we might decide whether the general (or quasi-general) equation has three independent constants...or four or more than four. Uncritically we might push the inquiry further, if there are more than four, but with rapidly increasing difficulties of various kinds, and with diminishing value of the results obtained, since the most simple results are the most valuable. Incidentally, such an investigation would enable us to distinguish those substances which are typical from those which are exceptional or 'abnormal'".
The following development in the field proceeded essentially the path that Gibbs had outlined, although his text did not influence further investigations. In particular, the fourth parameter foreseen by him, was introduced in the middle of 1950s in three possible variants, the most known of them being the 'acentric factor' [23]. It is to note that structure considerations were and are used to select groups of similar substances.
At last, structural models should be considered. They had also appeared in thermodynamics due to Gibbs. The first explicit manifestation of such an approach had been given by him in his models of interface layer (the 'dividing surface') and of nuclei of a new phase ('globular masses', or 'small globules') [24]. He based there, according the logic of his treatise, on 'macrostructure', but his main contribution to development of structure models was, certainly, statistical mechanics constructed for "the rational foundation of thermodynamics".
A. Rakovsky, who had been a participant and a witness of chemical thermodynamics evolution for about four decades, beginning from 1900s, made an interesting remark as to interrelations between thermodynamics and the kinetic theory: "Both Clausius and Gibbs adhered to the purity of lines in the two fields without their interference, but that became more difficult after investigations of Boltzmann and especially of Planck: the assimilation of elements of kinetic theories by thermodynamics becomes more and more persistent and advisable" [25].
The purity of lines could be easy kept in the last century when the structures variety of objects in chemical thermodynamics was not so enormous as at present, and the last was engaged into nearest and simplest objects - that was then enough. The radical change occurred, when quantum statistical calculations of heat capacities had been required after the Nernst’s heat theorem had been established. This topic was considered elsewhere [26]. The only elucidation here is that the intrusion of statistical mechanics ideas upon chemical thermodynamics had the general cause in its continuous broadening: the more became the variety of objects studied by chemical thermodynamics, the more became the variety of structures to be included into consideration.
Objects of modern chemical thermodynamics may be classified, as follows:
1. Particles: atoms, molecules, ions, radicals, transition complexes, clusters, macromolecules, micelles;
2. Phases: differing a/ by the aggregate state (crystalline, liquid-crystalline, amorphous, liquid, gaseous); b/ by the type of bonding (metallic, ionic, covalent, molecular); c/ by the number of components;
3. Interfaces and phase boundaries: charged and uncharged; two-phase (S-S, S-L, S-G, L-L, L-G, G-G); three-phase (S-S-S, S-S-L, S-S-G, S-L-L, L-L-L, L-L-G, L-G-G,...)',
4. Disperse systems: sols, emulsions, foams, aerosols, porous solids, ultra disperse solid media;
5. Complex systems, or various combinations of the previous classes 1-4.
Certainly, any chapter of chemical thermodynamics devoted to an object of the above list includes one or another structural model of the object. The general way of obtaining the corresponding thermodynamic functions is shown by statistical mechanics. This way stays almost impassable for the majority of real systems, so that at some distance from the ideal, that is from the derivation from the first principles, we must introduce some assumptions on values of parameters, or on forms of functions in general equations of statistical mechanics. It is to the point here Gibbs' another remark on his potentials: "In most cases, probably, we must content ourselves at first with finding out what we can about these functions without expecting to arrive immediately at complete expressions of them" [17]. That is why we have dozens of models in the same field, as, for example, in theories of solutions; here is even formed a special discipline called 'molecular thermodynamics’ [27].
Evaluating the whole picture one could notice that a counter movement occurs: the 'strict physics' and the 'empirical chemistry' go to meet each other, as well as it was a century ago - but to-day already at the level of statistical theory, not of the phenomenological. It is striking that the theoretic basis of the up-to-date chemical thermodynamics has been also determined by Gibbs.
References
1. J.W.Gibbs, Thermodynamic Works (In Russian), ed. V.K. Semenchenko, (Gostekhizdat, Moscow-Leningrad, 1950), p.14.
2. L. P. Wheeler, Josia Wtllard Gibbs: the history of a great mind, Yale Univ. Press, New Haven, 1951.
3. J. W. Gibbs, On the equilibrium of heterogeneous substances, Repertorlum der Mathematik, Hrsg. von L. Koenigsberger and G. Zeuner, 2 (Leipzig, 1879), 300-320.
4. Belbl. zu Wied. Ann., 4 (1880), 864-871.
5. A. Ya. Kipnis, A contribution to the history of the Glbbs' thermodynamlc theory (In Russian), Boпpocы истории ecтecтествознания и техники, 8 (1959) 127-132.
6. E. Garbor, J. C. Maxwell and thermodynamics, Amer. J. Phys. 37 (1969) 146-155.
7. A. Ya. Kipnis, B. E.Yavelov, Johannes Dlderlk van der Waals (In Russian), (Nauka Press, Moscow-Leningrad, 1985).
8. Jahresber. ueber Fortschrltte d. Chemle, (1876) 63.
9. A. Ya. Kipnis, An outline of the chemical thermodynamics formation (In Russian), Труды Института Истории Естествознания и Техники 35 (1961) 39-107.
10. V. V. Raman, The permeation of thermodynamics into nine¬teenth century chemistry, Indian J. Chem. 10 (1975) 18-37.
11. R. B .Dobrotin, Yu. I. Soloviev, J. H. van't Hoff (In Russian), Nauka Press, Moscow, 1977.
12. A. Ya. Kipnis, On the history of the chemical thermodynamics methods, Вопросы истории естествознания и техники, 27 (1969) 32-26.
13. J.W. Glbbs, The Collected Works, Longmans, 1928, vol.1, p.355.
14. Th. Belpaire. Note sur le second principe de la thermodynamique, Bull. de l'Acad. Roy. Belgique 34 (1872) 509-526.
15. J. C. Maxwell, Talt's ‘Thermodynamics', Nature, 17 (1878), 258.
16. G. Tamman, A contribution to the thermodynamics of one-component systems (In Russian), Журнал Русского Физико-Химического Общества, часть физическая, 44 (1912), 83-84.
17. See Ref. 2, p. 88-89.
18. See Ref. 13, p. 407-408.
19. Ibid. p. 425-434.
20. A.Б. Млодзеевский, The Geometric Thermodynamics (in Russian). Moscow Univ. Press, 1956, p.3.
21. See Ref. 13, p. 418.
22. C. Barus, Amer. J. Sci., 21 (1906), 461-462.
23. K.S. Pitzer et al, J. Amer. Chem. Soc., 77 (1955), 3433.
24. See Ref.13, p. 219, 253.
25. Дж. Партингтон, А. В. Раковский, A Course of Chemical Thermodynamics (in Russian), Moscow-Leningrad, 1932, p.11.
26. A. Ya. Kipnis, The heat theorem in the history of chemical thermodynamics, Вопросы истории естествознания и техники, 4(53) (1976) 42-47.
27. J. M. Prausnitz, Molecular Thermodynamics of Fluid-Phase Equililibrla, Prentice Hall, New York, 1969.
